One to One Functions are functions that have one domain and its own range. The range will not be the same for all domains (that will be considered as a many to one function.)
Examples of One-to-One Functions:
We can also conclude a one-to-one function if we inverse the function.
One to one function in graphs. If we want to conclude a function in a graph shown, we will do the vertical line test and the horizontal line test. The vertical line test determines if the graph is a function while the horizontal function determines if the function is a one-to-one function.
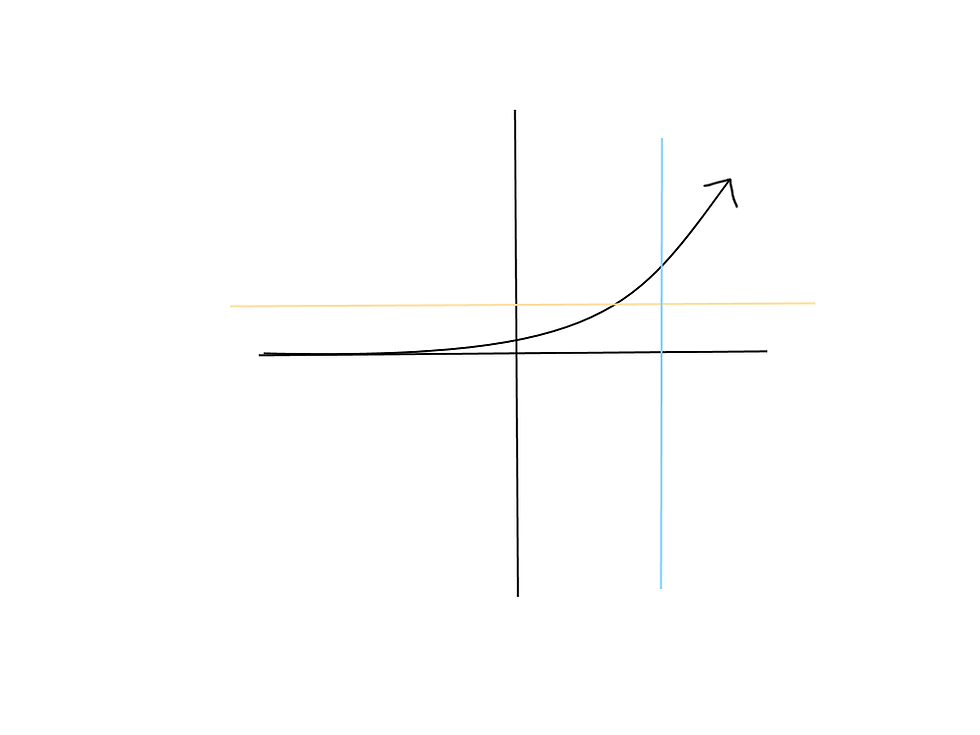
One to One Inverse Functions
Let the function f: A -> B be one to one function. Then the inverse of f denoted by f^-1 (if read, inverse of f) is a function with function B with range A.
Note: A function has an inverse function if and only if it is one to one.
To find the inverse function of a one-to-one function:
1. Write the function in form y = f(x).
2. Interchange the x and y variables.
3. Solve y in terms of x.
Example:
f(x) = 3x + 1
y = 3x + 1
x = 3y + 1
x – 1 = 3y
x – 1/3 = 3y/3
y = x – 1/3
If we invert y = x – 1/3, it will revert to y = 3x + 1. Just apply the same steps as before when we want to inverse y = x – 1/3.
Quadratic Functions (ex. f(x) = x^2 + 8x + 16) and functions that have absolute value (ex. f(x) = |x|) are not one to one inverse functions, since in their graphs, don’t represent the horizontal line test very well.
Comments